Electromagnetic levitation
Take 20 minutes to prepare this exercise.
Then, if you lack ideas to begin, look at the given clue and start searching for the solution.
A detailed solution is then proposed to you.
If you have more questions, feel free to ask them on the forum.
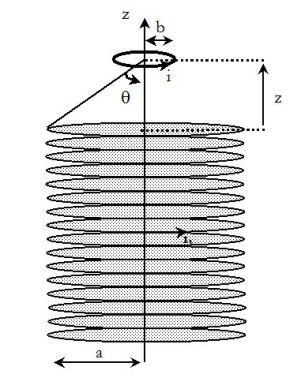
A long, half infinite, vertical solenoid of circular section (of radius
and made of
joined whorls by length unit) is travelled by a current intensity :
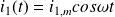
A circular coil made of
whorls of radius
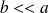, of résistance
, inductance
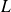and mass
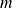is placed above the solenoid, distant from
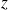to its end.
The position of the coil is marked by angle
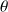
The magnetic field due to the solenoid at the center of the whorl is given by :
With :
Question
Determine the electric equation verified by the current
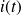induced in the coil.
We will write :
.
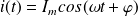Calculate the mutual induction of the two circuits and use complex notation to solve the electrical equation.
Suppose the field is uniform at the surface of the whorl. Its flux through the coil is :
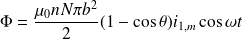
A mutual inductance between the whorl and the solenoid can be defined :
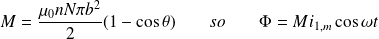
The electric equation of the coil is then :
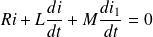
In a forced sinusoidal state :
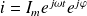
So :
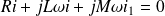
Hence :
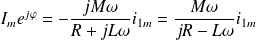
It can be deduced :
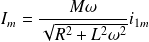
And :
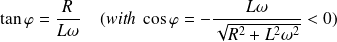
Question
Show that the radial magnetic field in the whorl can be written :
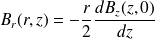
The magnetic field can be obtained by expressing the conservation of the magnetic flux.
Let's compute the radial component of the field.
Outside the
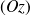axis, the magnetic field can be expressed :
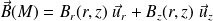
We can notice that the plane défined by the point M and
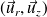is a anti-symetrical plane for the current distribution along the wire, so the magnetic field is in this plane and has no coordinate along
.
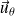Outside the axis, the magnetic field can be obtained by expressing the conservation of the magnetic flux :
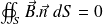
A small cylinder, centered on axis Oz, of height
and small radius
is used.
The flux, exiting through this closed surface must be equal to zero :
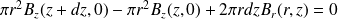
Thus :
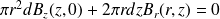
So :
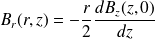
Question
Deduce the Laplace's force applied to the whorl.
Under which conditions is levitation possible ?
Is this possible equilibrium stable or unstable ?
Use the definition of the Laplace force and show that only the magnetic fied along
is usefull.
The Laplace's force (mainly vertical) is :
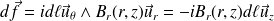
So :
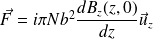
However :
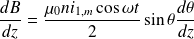
And :
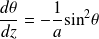(Use
)
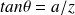Thus :
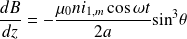
The force becomes :
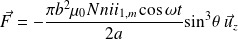
Hence :
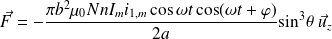
The mean value : (using
)
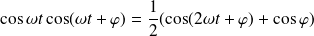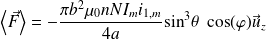
With :
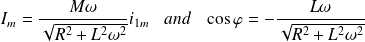
It comes :
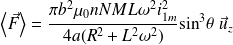
It is indeed a repulsive force (oriented upwards).
Right above the solenoid,
.
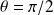Levitation is possible if the repulsion force is superior to the weight.
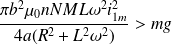
It is a stable equilibrium : if the mass goes up, the repulsion force diminishes and the mass goes back down.
Same if the mass begins by going down.
