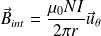Ampère's circuital law
Presentation of electricity and magnetism video (Référence : "Physique collégiale")
Fondamental :
Stokes' theorem, which we use without demonstration, is the equivalent of Ostrogradsky's theorem.
Stokes' theorem :
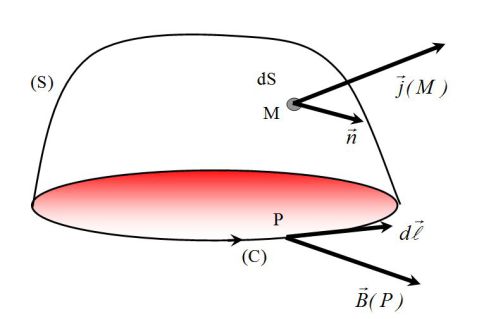
Let
 be an oriented closed loop and
be an oriented closed loop and
 a given surface which is supported by
a given surface which is supported by
 (as if it was a hat and
(as if it was a hat and
 was the extremity).
was the extremity).
The normal vector of the surface
 is oriented with the Right-hand rule.
is oriented with the Right-hand rule.
Stokes' theorem is :
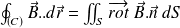
This theorem allows us to write Ampère's circuital law in its integral form.
Maxwell- Ampère equation in static regime is :
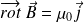
We compute the line integral of the magnetic field around a closed loop
 at a given moment. A surface
at a given moment. A surface
 is supported by
is supported by
 .
.
Let's use the Maxwell- Ampère equation :
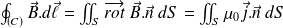
We recognize :
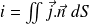
The intensity going through
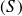 .
.
Hence Ampère's circuital law is :
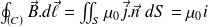
Attention : Flux and circulation of B (Ampère's circuital law)
The flux piercing through a closed surface of
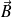 is equal to zero :
is equal to zero : 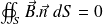
Ampère's circuital law :
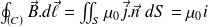
Méthode : Classic use of Ampere's circuital law
Ampère's circuital law helps computing easily a magnetic field in a highly symmetrical problem, especially in these must-know classic examples :
Infinite wire (without thickness) crossed by a current
 :
: 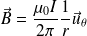
The cylindrical base is used.
Infinitely long solenoid, composed of
 whorls by length unit, crossed by a current
whorls by length unit, crossed by a current
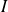 :
: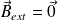
A JAVA animation by JJ.Rousseau on the field of a solenoid : click HERE
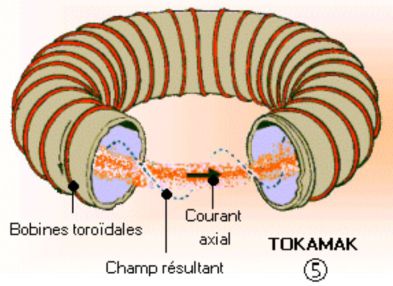
Magnetic field created by a torus (the one from the picture above), composed of
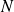 whorls crossed by current
whorls crossed by current
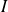 :
:
The cylindar base is used.
Simulation :
A JAVA animation by JJ Rousseau on the field of a torus : click HERE