Hall Effect

An electrical conductor under a voltage difference sees its carrier electrons set in motion.

The lesson about Ohm's local law specified the notations.

The electrical conductor is additionally placed in a magnetic field .

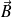Newton's second law applied to a charge carrier is expressed by :

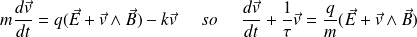
The following picture outlines the geometry of the conductor used : it is a parallelepiped rectangle of length .

The exterior magnetic field is .

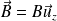The electric field created by the generator which sets charge carriers in motion is called .

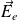In steady-state, electrons move with the velocity .

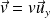In steady-state ( ) :

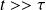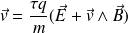
The magnetic term 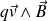is oriented as Ox axis. Thus an electric field appears, called the Hall field 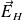such as :

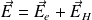
With :

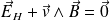
Thus, the speed of the carriers becomes :

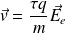
This Hall field is induced by the motion of electrons during a brief moment (length of transitory regime) towards side (2).

This creates a dissymmetry of charges, thus an electric field oriented from side (1) to side (2).

The Hall electric field is :

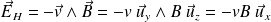
The Hall voltage 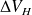is obtained by calculating the line integral around a loop of the field :

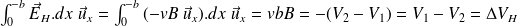
Thus :

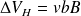
The intensity of the current is :

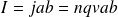
Consequently :

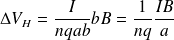
If 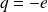(carrier electrons case, with 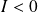on the picture) :

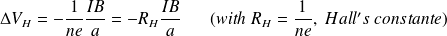
Hence we can see the Hall voltage is proportional to the magnetic field that is applied.

Hall probes can measure this voltage and deduce the value of the magnetic field.